Нас будет интересовать одна трактовка 4-го Предложения 7-ой книги "Начал":
http://www.px-pict.com/7/3/1/1/4/2/7/2/23.htmlиз "которого впоследствии развилась характерная для греческой математики теория отношений и пропорций".
... Два измерения позволяют естественным образом визуализировать расслоение пространства

элементарных звучий:
http://www.px-pict.com/9/6/8/2/2/3.htmlгде в качестве расслаивающего отображения выступает функция, реализуемая калькулятором из пункта 1 со страницы:
http://www.px-pict.com/10/4/4/1.htmlЯ буду использовать стандартную терминологию для расслоений, которая приведена, например, здесь:
http://www.px-pict.com/9/4/6/1/2/1.htmlhttp://www.px-pict.com/9/4/5.html-- Чт янв 21, 2016 23:53:58 --Значит, пространством расслоения будет множество всех упорядоченных пар натуральных чисел, а его базой -- множество все строк в алфавите из двух символов
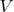
и
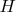
, о котором я уже писал здесь:
Чтобы записать “законы роста” из предыдущего поста в виде универсальных предложений, нужно спустить фигурирующие там строки символов в алфавите
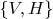
с мета-уровня на объектный уровень.
Для этого естественно расширить систему

, заставив действовать на ее множестве-носителе
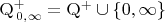
свободную полугруппу строк
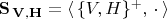
,
где
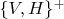
есть множество всех строк в алфавите
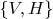
,

есть операция конкатенации строк (которую при записи термов будем, как правило, опускать).
Тогда мы могли бы сказать в духе Аристотеля, что две упорядоченные пары натуральных чисел пропорциональны тогда и только тогда, когда они имеют один и тот же антанаиресис.
http://www.px-pict.com/7/3/1/14/2/6/3/3.html