Ну, вот же мысля у меня была
http://dxdy.ru/viewtopic.php?t=3044:
Если есть квантовые компьютеры экспоненциально эффективнее классических, может есть суперструнные компьютеры экспоненциально эффективнее квантовых? А как насчёт промежуточных по эффективности компьютеров между квантовыми и классическими, а?
 http://www.physicsforums.com/archive/index.php/t-125043.html
http://www.physicsforums.com/archive/index.php/t-125043.html
Цитата:
Континуум-гипотеза: С точностью до эквивалентности, существуют только два типа бесконечных числовых множеств: счётное множество и континуум.
Аналогия вроде есть с мыслью выше?

Т.е. классические компьютеры - это счётные множества
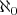
, квантовые компьютеры - континуум
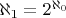
, струнные -
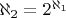
Цитата:
В 1963 году американский математик Паул Коэн доказал, что континуум-гипотезу нельзя ни доказать, ни опровергнуть.
Что это значит?

Значит в нашем реальном мире континуум гипотеза может быть как истинной так и ложной, вот я и хотел спросить, что изменится в нашем мире от того так это или нет.

А вот, что говорит
Скот Ааронсон:
There actually is a tenuous connection between quantum computing and set theory, which I'll touch on in the next lecture. To give a sneak preview, the connection is that
quantum mechanics applied to finite-dimensional systems (like qubits) seems like an interesting "intermediate" case between a continuous and a discrete theory. That is, it involves quantities (the amplitudes) that vary continuously, but that are not directly observable. In this way, it seems to avoid the "paradoxes" associated with the continuum in a way that other continuous physical theories do not.
Т.е. квантовые компьютеры промежуточны между счётным и континуумом.


 NP-complete Problems and Physical Reality
NP-complete Problems and Physical Reality
Can NP-complete problems be solved efficiently in the physical universe? I survey proposals including soap bubbles, protein folding, quantum computing, quantum advice, quantum adiabatic algorithms, quantum-mechanical nonlinearities, hidden variables, relativistic time dilation, analog computing, Malament-Hogarth spacetimes, quantum gravity, closed timelike curves, and "anthropic computing." The section on soap bubbles even includes some "experimental" results. While I do not believe that any of the proposals will let us solve NP-complete problems efficiently, I argue that by studying them, we can learn something not only about computation but also about physics.
 S. Aaronson. Is P Versus NP Formally Independent?
S. Aaronson. Is P Versus NP Formally Independent?
This is a survey about the title question, written for people who (like the author) see logic as forbidding, esoteric, and remote from their usual concerns. Beginning with a crash course on Zermelo-Fraenkel set theory, it discusses oracle independence; natural proofs; independence results of Razborov, Raz, DeMillo-Lipton, Sazanov, and others; and obstacles to proving P vs. NP independent of strong logical theories. It ends with some philosophical musings on when one should expect a mathematical question to have a definite answer.



