ok too for replace magnet and say it's f(1/d²), just a theorical study replace ball by point particle ;) At France, when I said that all people say: It's not possible ! even I say it's a theorical study ...
That is strange. Such kind of problems is very usual for theoretical physics. You could also say this is
theoretical model,
abstract model, or even
toy model.
By the way, on this forum we use LaTeX to write formulas. Using non-latin sybmols is unwanted. For example, you could just write in your text:
...say it's $f(1/d^2)$, just a...
and it would give
...say it's  , just a...
, just a...
For more examples and notations, you can look in
«Как набирать формулы?» and
«FAQ по тегу [ math ]».
With drawings I thought at first the pressure is not the same, but I'm not sure, I'm looking on internet, I asked at collegues and what I can say :
1/ It's not possible to calculate because it's too complex
2/ I contacted Comsol sofware, and for now it's not possible to simulate with the last software, nobody has done this type of simulation. A collegue said too it's not possible to simulate now. And without magnet only with simple electrostatic point particule !
3/ I don't find research study where I can have the result or even a study in this field, I'm not sure this study has done
4/ It's not possible to test easily, need a lot of magnets balls and if I want a near homogenous field I need a lot of small magnets, irons, very complex study, this is very difficult to build one ball so 1000 or more...
This all is correct, to some degree. Yes, the question is hard to give an
exact answer. Yes, it is hard even for numerical software, and it is hard to research experimentally. Yes, it is unlikely to find the research of
exactly this problem setting. But.
This
class of problems is very well-known in physics. It is called the
many body problem or
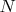 -body problem
-body problem (where
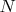
is considered large). It is usually impossible to give an
exact solution, but sometimes it is possible to find an
approximate answer, which is good enough for qualitative questions, and for quantitative ones at some precision.
For the systems of very many similar bodies, there is a powerful method of consideration, called
statistical physics. It gives
approximate answers, which becomes more accurate as the number of bodies becomes larger. And it is relatively easy to obtain these answers.
Also, it is useful to try to get the answer in the analytical form (as a formula). Playing with formulas is sometimes much more powerful than using numerical methos and software.
So, why I think the pressure is different ? because the black object change forces in each particule. For have the pressure in each point, I take a particule, I calculate without black objets all forces from all others particules, and like the geometry is different why the sum of forces will be equal ? Ok, there is lateral force that move particule a little for have only a perpendiculary force from black object but why this force must be the same in each point ?
Another problem: the shape of the recipient, here I drawn a circle (or sphere in 3d), but imagine something asymetric, in this case it will be very strange to have the same pressure in each point.
I think that easiest way to deal with the problem is to consider the statistical limit. That is, we imagine that the number of balls increases while the balls themselves become smaller (their repulsive forces also diminish in scale, that is, for example, instead of
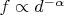
they become like
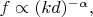
where
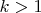
is the scale factor). Size of balls and their numbers are changed so as to keep the constant
density. Then we can go to the limit
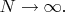
In this limit, the mass of balls starts to behave like some continuous media, that is, substance.
Now, would it become like a liquid or like a solid state substance? Solids resist to the sheer deformation. But you have drawn balls rather sparce, and it seems they won't resist if one tries to shift them by layers. So I think, these balls would be like a liquid, either viscous or not (that does not matter now). And then I recall the Pascal's law, that says that the pressure onto the object, immersed into the liquid, would be the same onto every point of the object, whatever size and shape it has.
And one last note:
3/ I don't find research study where I can have the result or even a study in this field, I'm not sure this study has done
3/ J'ai cherché au niveau recherches scientifiques et je n'ai rien trouvé, même pas quelque chose dans le domaine similaire
Actually, the search for scientific researches is not easy. It needs some practical skills, and if you don't have them, you can easily fail. Please consult your colleagues and teacher about how to perform this search more thoroughly and effectively. Here are some tips:
- search for the scientific papers in scientific journals and proceedings;
Google Scholar,
arXiv- know the right
keywords to use in the search, which describe the domain and concepts;
- make your search muli-stage. Look for review articles, which give you some basic knowledge about the domain, read them, make more clear idea of what you want, and then search further, based on that idea and new keywords (and maybe particular journals, authors, paper titles).



