Хотя это и совсем в стороне от обсуждаемой темы, но интересное мнение
sowa, которое обратило на себя моё внимание, и которое я хочу процитировать:
What is mathematics?Цитата:
Let me say only point out that mathematics has any value only as human activity. It is partially a science, but to a big extent it is an art. All proofs belong to the art part. They are not needed at all for applications of mathematics. If a proof cannot be understood by humans,... they have no value. Or, rather, their value is negative: a lot of human time and computer resources were wasted.
(Остальная часть поста - развёрнутые пояснения и комментарии к этому тезису, тоже интересные и крайне наглядные, но я не хочу перегружать данную тему цитированием офтопика.)
(Оффтоп)
Лично мой комментарий: в таком смысле, в любой science есть составляющая art: это та часть science, которая составляет идеи, и движет scientists к новым достижениям. Это необыкновенно важная часть science, но лично я не склонен отделять её от science вообще: без неё science мертва, и просто вырождается в collection of known facts. Например, две формулировки теории тяготения Ньютона,
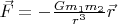
и

эквивалентны для вычислений, но совершенно не равнозначны для науки: они ведут к разным теоретическим модификациям и обобщениям. Они отличаются тем, что в вышеупомянутом обсуждении называлось conceptual framework и conceptual context.
По поводу алгебраических/аналитических областей, как я понял это, написал выше.
Да, спасибо, ваши пояснения почти проясняют пассаж Тао о КдФ для меня целиком. Видимо, неясными остаются только незначительные моменты. Я ещё перечитаю.
В той же дискуссии у Гауэрса упоминается изречение, приписываемое Манину: "доказательства важнее теорем, определения важнее доказательств".
Да, и неоднократно (и приводит его, в основном, тоже
sowa, так что в отрывке о слове “conceptual” к этому изречению идёт явная отсылка. Я хотел процитировать, но не дошли руки. Спасибо!
Также я встречал еще одно высказывание Манина: "за каждым определением стоит работа поколений математиков". Как-то так, не ручаюсь за дословность.
Ценно, хотя вариация того же самого.
А кристаллическая решетка здесь, имхо, просто метафора. С ней сравниваются алгебраические структуры, как нечто наиболее упорядоченное. И противопоставляется беспорядок и хаос других математических объектов.
Кажется, я понял, в чём дело. Я думал про "кристалл" с точки зрения его внешней формы - красивого многогранника - а вовсе не с точки зрения кристаллической решётки :-) В этом смысле, как эпитет this makes much more sense. Спасибо!
-- 11.06.2013 15:08:09 --Продолжение на ту же тему (похоже,
Vince Diesel заинтересовался) в посте
sowaThe conceptual mathematics vs. the classical (combinatorial) one.Опять, в качестве аннотации приведу только небольшую цитату:
Цитата:
It is not easy to explain how conceptual theorems and proofs, especially the ones of the level close to the one of Grothendieck work, could be at the same time more easy and more difficult at the same time. In fact, they are easy in one sense and difficult in another. The conceptual mathematics depends on – what one expect here? – on new concepts, or, what is the same, on the new definitions in order to solve new problems. The hard part is to discover appropriate definitions. After this proofs are very natural and straightforward up to being completely trivial in many situations. They are easy. Classically, the convoluted proofs with artificial tricks were valued most of all. Classically, it is desirable to have a most elementary proof possible, no matter how complicated it is.
A lot of efforts were devoted to attempts to prove the theorem about the distribution of primes elementary. In this case the requirement was not to use the theory of complex functions. Finally, such proof was found, and it turned out to be useless. Neither the first elementary proof, nor subsequent ones had clarified anything, and none helped to prove a much more precise form of this theorem, known as Riemann hypothesis (this is still an open problem which many consider as the most important problem in mathematics).
Остальная часть поста демонстрирует те же идеи на примере теоремы Стокса, и завершается ещё несколькими обобщающими словами.



