Связь Цилиндрических ортов с Декартовыми

[grandmix]

помогите пожалуйста разобраться. такая связь между Цилиндрических ортов с Декартовыми

вытекает откуда Или это просто определение ??

[grandmix]

вверх!

[svv]

(Оффтоп)

grandmix писал(а):

вверх!

Нельзя так, по правилам.

Нет, конечно, не просто определение. Базисные векторы полностью определяются такими двумя требованиями.

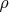
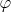
Первое требование определяет направление векторов: они являются касательными к координатным линиям (которые получаются, если одну координату менять, а остальные зафиксировать). В случае цилиндрических координат это радиусы (меняется только $\rho$), окружности (меняется только $\varphi$), ну, и вертикальные линии (меняется $z$).

Второе требование определяет длину векторов: при подходе, применяемом в Вашей книге, базисные векторы имеют единичную длину. В более серьёзной теории это не всегда так, но здесь мы этому следуем.

Конечно, чисто технически при расчете не обязательно надо прямо так непосредственно исходить из этих условий -- существуют удобные формулы. Вот как может выглядеть расчет.

Первый способ, "математический".
Находим вспомогательные векторы
$\tilde{\mathbf e}_{\rho}=\frac{\partial x}{\partial \rho}\mathbf e_x+\frac{\partial y}{\partial \rho}\mathbf e_y+\frac{\partial z}{\partial \rho}\mathbf e_z=\cos\varphi \;\mathbf e_x+\sin\varphi \;\mathbf e_y+0 \;\mathbf e_z$
$\tilde{\mathbf e}_{\varphi}=\frac{\partial x}{\partial \varphi}\mathbf e_x+\frac{\partial y}{\partial \varphi}\mathbf e_y+\frac{\partial z}{\partial \varphi}\mathbf e_z=-r\sin\varphi \;\mathbf e_x+r\cos\varphi\; \mathbf e_y+0\; \mathbf e_z$
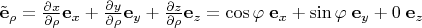
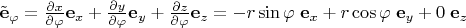
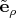
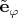
Они почти правильные, только не нормированы на единичную длину (в данном случае $\tilde{\mathbf e}_{\rho}$ сразу получился хорошим, но $\tilde{\mathbf e}_{\varphi}$ -- нет). Нормируем, получаем то, что в книге. Кстати, с точки зрения "серьёзной теории" эти промежуточные неединичные векторы и есть правильные базисные векторы в цилиндрической системе, и нормировать их не надо -- но я Вам этого не говорил.

Второй способ, "инженерный".
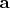
Имеется формула для разложения произвольного вектора $\mathbf a$ по ортонормированному базису, например, $\mathbf e_x,\mathbf e_y, \mathbf e_z$:
$\mathbf a = (\mathbf a \cdot \mathbf e_x) \mathbf e_x + (\mathbf a \cdot \mathbf e_y) \mathbf e_y + (\mathbf a \cdot \mathbf e_z) \mathbf e_z$
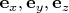
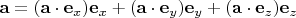
Применяем её к векторам $\mathbf e_{\rho}$, $\mathbf e_{\varphi}$:
$\mathbf e_{\rho} = (\mathbf e_{\rho} \cdot \mathbf e_x) \mathbf e_x + (\mathbf e_{\rho} \cdot \mathbf e_y) \mathbf e_y + (\mathbf e_{\rho} \cdot \mathbf e_z) \mathbf e_z$
$\mathbf e_{\varphi} = (\mathbf e_{\varphi} \cdot \mathbf e_x) \mathbf e_x + (\mathbf e_{\varphi} \cdot \mathbf e_y) \mathbf e_y + (\mathbf e_{\varphi} \cdot \mathbf e_z) \mathbf e_z$
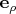
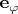
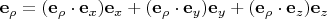
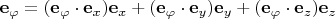
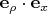
Так как все векторы в этой формуле единичные, скалярные произведения $\mathbf e_{\rho}\cdot \mathbf e_x$, $\mathbf e_{\rho}\cdot \mathbf e_y$ и т.д. равны просто косинусу угла между векторами. Остается как-то понять, исходя из конструкции цилиндрических координат, каковы же эти углы, и вместо скалярных произведений подставить их косинусы.

[grandmix]

svv
Большое спасибо!!!
Извиняюсь за офтоп, просто тема начала спускаться под толщами других тем. А вопрос всё никак не успокаивал. Теперь понятно, почему в большинстве литературы не приводится вывод формул, а даются сразу рабочие формулы .

[svv]

svv писал(а):

Базисные векторы полностью определяются такими двумя требованиями.
...
они являются касательными к координатным линиям

Чуточку уточню. Касательный вектор можно ещё провести "по" координатной линии и "против" -- перемена его направления на противоположное сохранит касательность. А чтобы совсем однозначно определялся, условились, что он смотрит в сторону возрастания соответствующей координаты, а не убывания.