легко, в том же Голдстейне об этом написано
Почитал Голдстейна. Ну такие "вариационные принципы" действительно иногда формулируют в учебниках по неголономным системам. Только эти принципы по сути своей отличны от принципа Гамильтона, который здесь обсуждается. Отличие состоит в том, что такие "вариационные принципы" для неголономной механки нельзя представить в виде: "предположим, что функция
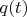
является экстремалью функционала
![$F[q(\cdot)]$ $F[q(\cdot)]$](https://dxdy-04.korotkov.co.uk/f/b/c/5/bc5a281d33ba0f2154886bec2864042982.png)
тогда
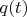
-- закон движения данной механической системы". С точки зрения вариационного счисления, у Голдстейна это вообще не вариационный принцип: нет функционала, который варировали бы.
Вот над этим Вам еще будет полезно подумать.
[A.M. Bloch: Nonholonomic mechanics and control. Springer]:
"Variational Nonholonomic Equations. It is interesting to compare
the dynamic nonholonomic equations, that is, the Lagrange–d’Alembert
equations with the corresponding variational nonholonomic equations. The
distinction between these two different systems of equations has a long and
distinguished history going back to the review article of Korteweg [1899]
and is discussed in a more modern context in Arnold, Kozlov, and Neishtadt
[1988]. (For Kozlov’s work on vakonomic systems see, e.g., Kozlov [1983]
and Kozlov [1992]).8 The upshot of the distinction is that the Lagrange–
d’Alembert equations are the correct mechanical dynamical equations,
while the corresponding variational problem is asking a different question,
namely one of optimal control.
Perhaps it is surprising, at least at first, that these two procedures give
different equations. What, exactly, is the difference in the two procedures?
The distinction is one of whether the constraints are imposed before or
after taking variations. These two operations do not, in general, commute."



