Производная от тета-функции

[PHT]

Функция $f\left( x \right)$ в точке $0$ претерпевает разрыв величиной $2h$.
Можно ли это описать с помощью тета-функции Хевисайда следующим образом:
$f\left( x \right) = f_1 \left( x \right) - h\theta \left( { - x} \right) + h\theta \left( x \right)$?
где $f_1 \left( x \right)$ - непрерывная функция.
А производная от нее:
$f'\left( x \right) = f'_1 \left( x \right) + 2h\delta \left( x \right)$?

Проверьте правильность рассуждений, пожалуйста.

Раз функция $\theta \left( { - x} \right)$ убывает, значит производная от нее должна быть отрицательной. Верно ли это утверждение в применении к обобщенным функциям?

[CowboyHugges]

PHT в сообщении #237835 писал(а):

Можно ли это описать с помощью тета-функции Хевисайда следующим образом:
$f\left( x \right) = f_1 \left( x \right) - h\theta \left( { - x} \right) + h\theta \left( x \right)$?

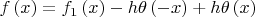
Как-то сомнительно, возьмите например:

$$f(x) = \left \{ \begin{array}{11} \sin{x} & x < 0 \\ \cos{x} & x >0 \end{array} \right. $$

PHT в сообщении #237835 писал(а):

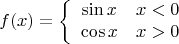
А производная от нее:
$f'\left( x \right) = f'_1 \left( x \right) + 2h\delta \left( x \right)$?

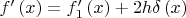
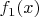
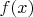
А вот это верно. Ну разве что для поборников строгости надо дописать, что исходная $f_1(x)$ гладкая и $f(x)$ почти всюду равна $f_1(x)$

PHT в сообщении #237835 писал(а):

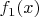
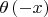
Раз функция $\theta \left( { - x} \right)$ убывает, значит производная от нее должна быть отрицательной. Верно ли это утверждение в применении к обобщенным функциям?

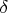
А что такое "отрицательная обобщенная функция" в общем случае. Например функция $\delta$ она отрицательная? Да и тета-функция она не совсем "убывает", она, если хотите, скорее "не возрастает"

[ewert]

PHT в сообщении #237835 писал(а):

Верно ли это утверждение в применении к обобщенным функциям?

Во-первых, не "убывает", а "невозрастает". Во-вторых, не то чтобы верно или неверно, а попросту бессмысленно: обобщённая функция -- не есть функция, и для неё это понятие просто не применимо.

[ИСН]

Устранимый разрыв - это вообще не разрыв, и не надо тут ничего - - -

[Хорхе]

Ну почему же, для обобщенной функции понятие неотрицательности вполне естественно: она неотрицательна тттк ее спаривание с любой неотрицательной основной функцией неотрицательно. И дельта-функция неотрицательна, конечно же.