Но Вы же поняли, что я хотел сказать.
Конечно. И я действительно был неправ в своих рассуждениях. Решил завернуть их в тег ошибки, чтоб не удалять целиком из последующего полилога (совсем не нужные глупости удалил).
Я теперь не могу найти, где прочитал то, что сбило меня с толку с этими codomain. Зато нашёл вот цитату, в которой как-то объясняется, как это можно понимать. А то обычные определения с упорядоченными парам или графиками этого понимания не дают. Насчёт аналогий с пустой функцией -- это всё было мимо.
англовики про альтернативы определения функций писал(а):
If a function is defined as a set of ordered pairs with no specific codomain, then
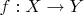
indicates that

is a function whose domain is
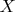
and whose image is a subset of
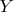
. This is the case in the ISO standard.
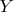
may be referred to as the codomain but then any set including the image of

is a valid codomain of

. This is also referred to by saying that "

maps
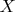
into
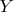
". In some usages
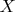
and
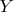
may subset the ordered pairs, e.g. the function

on the real numbers such that
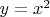
when used as in
![$f:[0,4]\to [0,4]$ $f:[0,4]\to [0,4]$](https://dxdy-02.korotkov.co.uk/f/9/f/1/9f1188af818029892b20b705ba67f68d82.png)
means the function defined only on the interval
![$[0,2]$ $[0,2]$](https://dxdy-02.korotkov.co.uk/f/5/1/b/51b74976bf5bf6412f174f5ee6f4449482.png)
.



