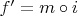На пальцах это так:

загоняет некоторые точки, напирмер
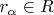
в одну и ту же точку, скажем
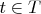
. Как Вы знаете, разные
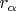
в этой совокупности отличаются на элемент из
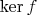
. Отображение факторизации "слепляет" такие точки в одну. Теперь можно рассмотреть отображение
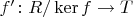
, отображающая кажду точку, полученную из слепления совокупности
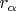
в соответствующую этой совокупности точку
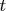
. Такое отображение будет, очевидно, мономорфизмом.
Точно так же рассуждая, строят каноническое разложение любого гомоморфизма
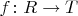
на эпиморфизм, изоморфизм и мономорфизм

Здесь

— это факторизация, а
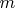
— естественное вложение. В этой схеме