Differential operator and Fourier Transform

[Bridgeport]

Could anyone help?!

I have nonzero differential operator with constant coefficients

$$Tf=\sum_{i=0}^{n} a_iD^if$$

. It acts on the space of smooth functions vanishing at infinity (over the real line - for simplicity, the problem could be also stated for partial differential operator). I need to show that Tf = 0 => f=0. There is a hint to use Fourier Transform. I have no idea how to use the hint.

Any input is greatly appreciated!

Spasibo

[незваный гость]

Apply FT to the $T f$. You should be able to calculate it explicitly thru $F$ (FT of $f$). Compare your result with FT of 0.

[Bridgeport]

незванный гость писал(а):

:evil:
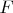
Apply FT to the $T f$. You should be able to calculate it explicitly thru $F$ (FT of $f$). Compare your result with FT of 0.

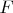
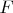
I am not totally familiar with notation. What does capital $F$ in the following expression mean $F$ (FT of $f$) ?

I tried to computer FT of the $Tf$. Well, for the first derivative I can apply integration by parts, to get at least something, but this trick does not work for the second derivative. (since $f'$ might not be vanishing at infinity)

In addition, FT of $f$ might not even exist by itself.

[незваный гость]

Bridgeport писал(а):

I am not totally familiar with notation. What does capital $F$ in the following expression mean $F$ (FT of $f$) ?

$F$ here is result of the Fourier Transform applied to $f$ (I tried to clarify it by phrase in parenthesis).

I'd like to think on the rest of your questions. The solutions for $ T f = 0 $ are well know linear combinations of compex exponents, but known of them can be said to vanish at infinity.

[Bridgeport]

Thank you for the clarifications!

I also want to add that problem would be much easier if our class of functions was Schwars class (i.e. vanishing faster than any polynomial )or $L^2 $, but in case of infinitely differentiable vanishing at infinity $C_0^\infty $ I cannot get differentiation by parts to work on the second and higher order derivatives to get relations between FT and derivatives. But even if I could, I still don't see the way how to proceed futher.

Spasibo!

[shwedka]

Bridgeport писал(а):

Thank you for the clarifications!

I also want to add that problem would be much easier if our class of functions was Schwars class (i.e. vanishing faster than any polynomial )or $L^2 $, but in case of infinitely differentiable vanishing at infinity $C_0^\infty $ I cannot get differentiation by parts to work on the second and higher order derivatives to get relations between FT and derivatives. But even if I could, I still don't see the way how to proceed futher.

Spasibo!

You must remember that a function in $C_0^\infty $ has all derivatives in the same class. Therefore you can apply Fourier to all derivatives and integrate by parts to get
$F(\sum C_k (d/dx)^k u)(\xi)=\sum(C_k (i\xi)^k)(Fu)(\xi)$
If this is zero, so $(Fu)(\xi)$ must be zero everywhere where the polynomial $\sum(C_k (i\xi)^k)$ is not zero. This means that $(Fu)(\xi)$
is zero everywhere with exception of a finite set of points, and a smooth function can be such only if it is zero.

[Bridgeport]

Ogromnoe spasibo!

Here is one more question. We have that $(Fu)(\xi)$ is a smooth function, which is zero everywhere. How can I use it to conclude that $u(x)$ is zero function? That was my originaly problem.

[shwedka]

Apply the inverse Fourier.
$F^{-1}F u=u,\; F^{-1}(0)=0$

[Bridgeport]

So far, I only know that fourier inverse is going to return original function, only in Schwars class and in $L^2$, (since Schwars class in dense in $L^2$). Do you think it is possible to get: $\hat{u}\check\ = u$, if $u \in C^\infty_0$?

[shwedka]

Think a little and you find that $C_0^\infty\subset\mathcal{S}$ (Schwartz)

[Bridgeport]

I guess my definition of Schwartz class is slighly different http://en.wikipedia.org/wiki/Schwartz_space
(functions and their derivatives in Schwartz class decrease rapidly to zero)


I would rather have:$\mathcal{S}\subset C_0^\infty\ $

[shwedka]

Write the condition of tending to zero as an inequality and check that the functions with compact support satisfy this inequality.
How do you understand
$C_0^\infty$??????

[Bridgeport]

$C^\infty_0 $ - I understand as vanishing at infinity, not as compactly supported.

$C^\infty_c $ - is compactly supported.

[shwedka]

In this case you contradict the usual notations.
what does 'vanishing at infty 'mean, exactly!!

[Bridgeport]

Here is definition that I use:

http://en.wikipedia.org/wiki/Vanish_at_infinity

The same defition and notation is used in Rudin "Real and Complex Analysis"