Кусочно-непрерывная функция и Липшицевость

[Arastas]

Добрый день!

Читаю одну книгу и прошу помощи с непонятным отрывком. Чтобы ничего не переврать, вот отрывок на языке оригинала:
Consider
$\dot{x}=A(t)\,x, \quad x(t_0)=x_0\qquad(1)$
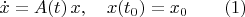
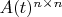
where $A(t)\inR^{n\times n}$ is a piecewise continuouse function belonging to $L_{\infty\,\varepsilon}$.
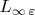
Далее идут некоторые определения, а затем
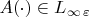
Note that linear systems with $A(\cdot)\in L_{\infty\,\varepsilon}$ automatically satisfy the Lipschitz condition over any finite time interval, so that the solutions of (1) are unique on any time interval.

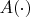
Мне не понятно - если $A(\cdot)$ - кусочно-непрерывная, то она ведь допускает разрывы на границах интервалов, так? Как она тогда может быть Липшицевой? Объясните, пожалуйста.

[Otta]

Arastas в сообщении #844123 писал(а):

Мне не понятно - если $A(\cdot)$ - кусочно-непрерывная, то она ведь допускает разрывы на границах интервалов, так? Как она тогда может быть Липшицевой?

Не она липшицева. Система удовлетворяет условию Липшица.

[Arastas]

Я, к сожалению, не понимаю. Система - в смысле, что правая часть диф. уравнения? Разве она Липшицева, если $A(\cdot)$ может быть разрывной?

[Otta]

Вы теорему Коши о существовании и единственности решения знаете в этой формулировке? дальше ведь на нее ссылаются. Найдите, все равно понадобится, заодно найдете и условие липшицевости для ОДУ (системы ОДУ).

[Dan B-Yallay]

Arastas в сообщении #844150 писал(а):

Разве она Липшицева, если $A(\cdot)$ может быть разрывной?

Вам намекают, что (при $0<t<T$) матрица $A(t)$ (даже если разрывна) - она ограничена сверху в некотором смысле.
Вот это ограничение и используется в виде множителя $L :$ Липшицево отображение

[Otta]

Dan B-Yallay
Ему намекают, что условие липшицевости для системы выглядит иначе и выписывается по совсем другой переменной, чем он думает.

[Dan B-Yallay]

он по $t$ проверятет Липшицевость?

[Otta]

Именно. И недоумение его понятно: ведь липшицева обязана быть непрерывной безо всяких "кусочно". ))

[Arastas]

Хорошо. Имеем $\dot{x}=f(t,x)$, где $f(t,x)=A(t)\,x$. Так как $A(t)$ ограничена, то функция $f(t,x)$ является Липшицевой по аргументу $x$, с этим разобрался, спасибо.

Однако далее я нахожу несколько различающихся формулировки теоремы о существования и единственности решения, но они все предполагают и Липшицевость по $x$, и непрерывность $f(t,x)$ по всем аргументам. В моем случае $f(t,x)$ не является непрерывной по $t$, только кусочно-непрерывной. Какие ограничения это накладывает на существование и единственность решения?

Спасибо за помощь.

[Oleg Zubelevich]

Arastas в сообщении #844189 писал(а):

Однако далее я нахожу несколько различающихся формулировки теоремы о существования и единственности решения, но они все предполагают и Липшицевость по $x$, и непрерывность $f(t,x)$ по всем аргументам. В моем случае $f(t,x)$ не является непрерывной по $t$, только кусочно-непрерывной. Какие ограничения это накладывает на существование и единственность решения?

это накладывает условия только на гладкость решения, см также условия Каратеодори