Тригонометрическое у-е

[kda_ximik]

Есть ур-ние:
$2\sin^3x-\sin^2x+2\sin{x}\cos^2{x}-\cos^3x=0$
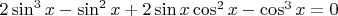
1) Сначала попробовал так: взял один "кибический синус" и объединил с "кубическим" косинусом и раскрыл разность кубов:
$(\sin{x}-\cos{x})(\sin^2x+\sin{x}\cos{x}+\cos^2x)+\sin^3x-\sin^2x+2\sin{x}\cos^2{x}=0$

$(\sin{x}-\cos{x})(1+\sin{x}\cos{x})+\sin^3x-\sin^2x+2\sin{x}\cos^2{x}=0$

$(\sin{x}-\cos{x})(1+\sin{x}\cos{x})+\sin{x}(\sin^2x-\sin{x}+2\cos^2{x})=0$

$(\sin{x}-\cos{x})(1+\sin{x}\cos{x})+\sin{x}(2-\sin^2x-\sin{x})=0$
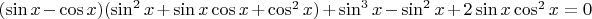
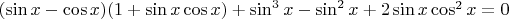
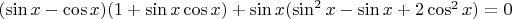
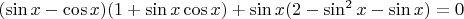
В правой скобке можно, конечно, разложить квадратный трехчлен, но мне это особо не помогло. Дальше не знаю куда двигаться

2) пробовал второй вариант: вынести за скобку $\sin{x}$ и $\cos^2x$.
$\sin{x}(2\sin^2{x}-\sin{x}+2\cos^2{x})+\cos^2{x}(\sin{x}-\cos^2{x}=0)$

$\sin{x}(2-\sin{x})+\cos^2{x}(\sin{x}-\cos^2{x})=0$
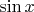
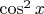
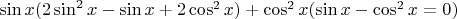
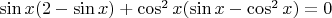
И тут дальше не знаю что делать...

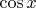
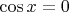
3) поделить на $\cos{x}$ не можем, т.к., если вдруг $\cos{x}=0$, то останется уравнение, имеющее конкретную серию корней
$2\sin^3{x}-\sin^2{x}=0$

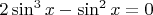
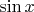
деление на $\sin{x}$, на мой взгляд, ни к чему хорошему не приводит...

Так что не знаю как дальше
Надеюсь, простых арифметических ошибок в вычислениях нет.

[nnosipov]

Получается плохое уравнение 6-й степени относительно тангенса. Нет ли опечатки в условии?

[kda_ximik]

Проверил - ошибок нет.

[nnosipov]

Я имел в виду опечатку в задачнике. Ответ там есть?

[kda_ximik]

Я понял, что речь идет о задачнике.
С собой книжки у меня сейчас нет, но помню, что ответ выражается через тангенс. Если не ошибаюсь $-\arctg2+\pi${k}

[TOTAL]

kda_ximik в сообщении #835462 писал(а):

С собой книжки у меня сейчас нет, но помню, что ответ выражается через тангенс. Если не ошибаюсь $-\arctg2+\pi${k}

Подстановкой проверьте, яляется ли ответ ответом.

[nnosipov]

kda_ximik в сообщении #835462 писал(а):

Если не ошибаюсь $-\arctg2+\pi${k}

Нет, это точно не ответ.

[kda_ximik]

ответ к задаче: $-\arctg\frac{1}{2}+\pi{k}$

[Алексей К.]

Не, это к какой-то другой задаче ответ.

[nnosipov]

kda_ximik в сообщении #835493 писал(а):

ответ к задаче: $-\arctg\frac{1}{2}+\pi{k}$

Тоже не подходит. Судя по форме ответа, во втором слагаемом левой части уравнения пропущен сомножитель $\cos{x}$ с подходящим коэффициентом.

[Евгений Машеров]

Коэффициент единица вполне подходит...

-- 12 мар 2014, 10:09 --

(Если, конечно, в ответе перед тангенс стоит тире, а не минус...)

[Евгений Машеров]

(Оффтоп)

Вообще-то при словах у.е. мне вспомнились времена, когда так доллары обозначали. "Тригонометрический бакс"...