Активный эксперимент

[UndeadBlow]

Всем привет.
В общем в институте запарился с одним пунктом из работы, не могу сам разобраться, поскольку преподавателя тяжело отловить. Если кто-то поможет разобраться и сделать - буду очень благодарен. Я уже 2 дня не могу разобраться.
Итак.

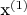
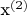
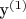
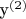
Есть две исходные независимые выборки {x}^{(1)}, {x}^{(2)}, {y}^{(1)}, {y}^{(2)}, соответственно, иксы - входные воздействия (факторы), игрики выходные (отклики).
Работая, большая, но вызвал проблему последний пункт:
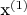
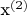
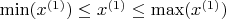
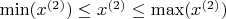
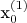
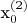
1. В области изменения факторов {x}^{(1)} и {x}^{(2)}, то есть \min({x}^{(1)})\leq {x}^{(1)}\leq \max({x}^{(1)}), \min({x}^{(2)})\leq {x}^{(2)} \leq \max({x}^{(2)}), выбрать исходную точку {x}^{(1)}_0, {x}^{(2)}_0 (нулевой уровень).
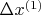
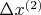
2. Выбрать шаги варьирования факторов (\Delta {x}^{(1)} и \Delta x^{(2)}), провести полный факторный эксперимент 1-го порядка, определить коэффициенты регрессии y` = a`_0 + a`_1 \cdot x^{(1)} + a`_2 \cdot x^{(2)} + a`_12 \cdot x^{(1)} \cdot x^{(2)}
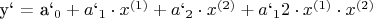
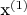
3. Осуществить просмотр факторного пространства по направлению оценок градиента функции y` по координатам {x}^{(1)} и x^{(2)}.
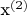
4. Выявить область, подозрительную на экстремум.
5. Принять координаты точки, в которой обеспечивается экстремум функции на найденном направлении, за нулевой уровень и повторить эксперимент.
6. Сделать выводы о характере поверхности отклика и о расположении экстремума функции y.
7. Если по результатам выполнения 1-5 нельзя сделать вывод о характере поверхности отклика и о нахождении экстремума функции y, необходимо повторить 1-5, выбрав другую исходную точку.

Формулы:
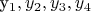
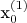
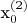
y_1, y_2, y_3, y_4 - По идее 4 точки вокруг выбранной ({x}^{(1)}_0, {x}^{(2)}_0) на расстоянии от нее 10% расстояния от оси. Нахожу через:
y({x}^{(1)} - {x}^{(1)} \cdot 0.1, {x}^{(2)} - {x}^{(2)} \cdot 0.1)
y({x}^{(1)} - {x}^{(1)} \cdot 0.1, {x}^{(2)} + {x}^{(2)} \cdot 0.1)
y({x}^{(1)} + {x}^{(1)} \cdot 0.1, {x}^{(2)} - {x}^{(2)} \cdot 0.1)
y({x}^{(1)} + {x}^{(1)} \cdot 0.1, {x}^{(2)} + {x}^{(2)} \cdot 0.1),
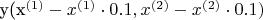
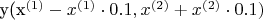
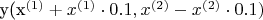
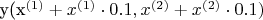
где y - функция, аппроксимирующая исходные данные (y = k_1 \cdot y^{(1)} + k_2 \cdot y^{(2)}

a_0 = \frac{1}{4} \cdot \sum_{j=1}^{4}y_j
a_1 = \frac{1}{4} \cdot \sum_{j=1}^{4}y_j \cdot {x}^{(1)}
a_2 = \frac{1}{4} \cdot \sum_{j=1}^{4}y_j \cdot {x}^{(2)}
{a}_{12} = \frac{1}{4} \cdot \sum_{j=1}^{4}y_j \cdot {x}^{(1)} \cdot {x}^{(2)},
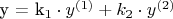
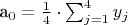
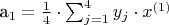
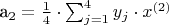
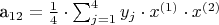
где
x^{(1)} | x^{(2)}
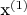
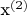
-1 | -1
-1 | +1
+1 | -1
+1 | +1
, ибо полный факторный эксперимент.

Шаги:
\Delta x^{(1)} \cdot k = a_1 \cdot \Delta x^{(1)} \cdot k
\Delta x^{(2)} \cdot k = a_2 \cdot \Delta x^{(2)} \cdot k

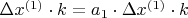
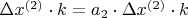
Уф, с теорией все. Теперь, что я делаю:
x^{(1)} | x^{(2)}
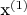
0.55 | 12.8
0.70 | 11.2
0.95 | 6.4
0.65 | 0.8
0.25 | 4.0
0.30 | 14.4
0.20 | 16.0
0.75 | 8.8
0.85 | 2.4
1.00 | 10.4
0.60 | 3.2
0.05 | 15.2
0.10 | 9.6
0.90 | 5.6
0.40 | 12.0
0.15 | 4.8
0.50 | 13.6
0.35 | 1.6
0.45 | 7.2
0.80 | 3.2

Аппроксимирующая функция:
y = 0.134 + 0.808 \cdot x1 + 1.0422 -0.29968 \cdot x2 + 0.026039 \cdot x2^{2} -0.00013699 \cdot x2^{3} -0.000030884 \cdot x2^{4}

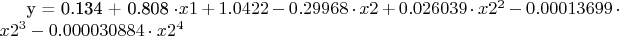
Выбираю точку x^{(1)} = 0.25, x^{(2)} = 4.0:
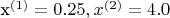
(Скрипт на Пайтоне по формулам выше)

Enter x1: .25
Enter x2: 4
Points around (0.25, 4.0):
Y (0.225, 3.6) = 0.605038708506
Y (0.225, 4.4) = 0.520278064154
Y (0.275, 3.6) = 0.645438708506
Y (0.275, 4.4) = 0.560678064154
In this point: a0 = 0.58285838633, a1 = 0.0202, a2 = -0.042380322176
Y` = 0.418387097626
Step /\x1` = a1(0.0202) * x1(0.25) * step(0.1) * k(1) = 0.000505
Step /\x2` = a2(-0.042380322176) * x2(4.0) * step(0.1) * k(1) = -0.0169521288704

С полученными шагами выполняю движение по осям, вычисляя на каждом шаге значение y, до тех пор, пока не выйдем за границы плоскости:

x1 = 0.25, x2 = 4.0, Y = 0.579430336
x1 = 0.250505, x2 = 3.98304787113, Y = 0.581638917442
x1 = 0.25101, x2 = 3.96609574226, Y = 0.583859834322
x1 = 0.251515, x2 = 3.94914361339, Y = 0.586093104996
x1 = 0.25202, x2 = 3.93219148452, Y = 0.588338747759
...
x1 = 0.333325, x2 = 1.20289873638, Y = 1.12241633752
x1 = 0.33383, x2 = 1.18594660751, Y = 1.12686362386
x1 = 0.334335, x2 = 1.16899447864, Y = 1.13132544618
x1 = 0.33484, x2 = 1.15204234977, Y = 1.13580181272
x1 = 0.335345, x2 = 1.1350902209, Y = 1.14029273169
x1 = 0.33585, x2 = 1.11813809203, Y = 1.14479821122
x1 = 0.336355, x2 = 1.10118596316, Y = 1.14931825937
x1 = 0.33686, x2 = 1.08423383429, Y = 1.15385288417
x1 = 0.337365, x2 = 1.06728170542, Y = 1.15840209356
x1 = 0.33787, x2 = 1.05032957655, Y = 1.16296589543
x1 = 0.338375, x2 = 1.03337744768, Y = 1.1675442976
x1 = 0.33888, x2 = 1.01642531881, Y = 1.17213730784
x1 = 0.339385, x2 = 0.999473189939, Y = 1.17674493387
x1 = 0.33989, x2 = 0.982521061069, Y = 1.18136718331
x1 = 0.340395, x2 = 0.965568932198, Y = 1.18600406376
x1 = 0.3409, x2 = 0.948616803328, Y = 1.19065558274
x1 = 0.341405, x2 = 0.931664674458, Y = 1.1953217477
x1 = 0.34191, x2 = 0.914712545587, Y = 1.20000256605
x1 = 0.342415, x2 = 0.897760416717, Y = 1.20469804512
x1 = 0.34292, x2 = 0.880808287846, Y = 1.20940819218
x1 = 0.343425, x2 = 0.863856158976, Y = 1.21413301447
x1 = 0.34393, x2 = 0.846904030106, Y = 1.21887251912
x1 = 0.344435, x2 = 0.829951901235, Y = 1.22362671323
x1 = 0.34494, x2 = 0.812999772365, Y = 1.22839560383

В общем, с этого момента я не понимаю ничего. Локальных экстремумов не видать, только увеличение значений, что делать не знаю. В общем, помогите, пожалуйста.

[Deggial]

Пост поправил:
1. Код и лог оформлены тегом code.
2. Неравенства $\leq$ пишутся \leq.
3. Минимумы и максимумы пишите со слэшем: \min, \max.
4. Формулы просто обрамляйте долларами - тег math проставится сам.

[Aritaborian]

«По-русски» неравенства пишутся так: \leqslant, \geqslant.

[provincialka]

Aritaborian, это все изыски. Особенно излишние, когда набираешь с телефона и вечно промахиваешься мимо кнопочек.

[arseniiv]

(О формулах.)

Так и тот код лишь для справки.

Вот здесь некоторые пишут вероятность как простую $P$, некоторые как sans serif $\mathsf P$, а некоторые, может быть, как $\mathrm P$. Вряд ли все выберут один вариант и станут использовать только его, какие бы веские причины за него не предлагали.

Хотя не все и не всегда пишут с телефона.

[Aritaborian]

provincialka, это не изыски, а традиционное в наших краях написание. Раз уж мы так пишем на доске и в тетради, можно найти в себе силы писать точно так же в Сети.
Кстати, почему бы не внести в форумный $\TeX$-движок соответствующую автозамену?