It is a school exercise about drawing the graphic of the function.
Then you should draw graphs of
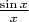
and
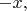
and then find their intersection points. Or, of
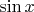
and
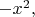
as was proposed above.
What is numerically more exactly?
Numerical solution can be done with a number of methods, for example:
1. Start with some point, e. g.
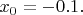
2. Calculate a
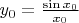
from it.
3. Assign
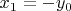
to be a next approximation.
Repeat the steps 2 & 3 several times, proceeding to values
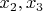
and so on. If this process converges, stop when you reached the target precision, i. e.
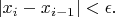
There can be 'bad initial guesses' which lead to divergence, in such case try some other initial guess.



