This part is concerned with the effect of a gravitational field on the propagation of light in space.
(a) A photon emitted from the surface of the Sun (mass

, radius

) is red-shifted. By assuming a rest-mass equivalent for the photon energy, apply Newtonian gravitational theory to show that the effective (or measured) frequency of the photon at infinity is reduced (red-shifted) by the factor
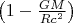
(b) A reduction of the photon's frequency is equivalent to an increase in its time period, or, using the photon as a standard clock, a dilation of time. In addition, it may be shown that a time dilation is always accompanied by a contraction in the unit of length by the same factor.
We will now try to study the effect that this has on the propagation of light near the Sun. Let us first define an effective refractive index
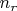
at a point

from the centre of the Sun. Let
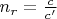
where

is the speed of light as measured by a co-ordinate system far away from the Sun's gravitational influence
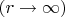
, and
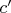
is the speed of light as measured by a co-ordinate system at a distance

from the centre of the Sun.
Show that
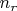
may be approximated to:
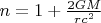
Моя попытка решения:
(a) Чтобы узнать эффективную массу фотона, приравняем его энергию к релятивистской энергии

а значит
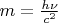
Закон сохранения энергии
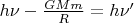
откуда
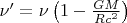
(b)
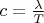
а значит, согласно тексту (время уменьшается а расстояние увеличивается, и наоборот),
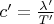
Из того же сохранения энергии получаем что
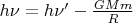
а значит
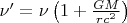
откуда
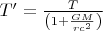
Откуда получим что
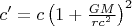
против
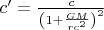
в ответе.
В чем заключается ошибка?



