The sum of energy in an isolated device

[Ludovic]

Hi,

I would like to verify the sum of energy of this isolated device:



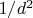
The half disk has a lot of small balls inside. No friction. The mass of the ball is 0 (theory). There are springs that attract balls with the law $1/d^2$ to have the pressure on the walls like gravity can do with water. The mass of the spring is 0. Each spring is attached from the red center to a ball. All the device turns around the red center. The center of the half disk turns too around the red center but it is the black arm that take the black center. Like the centers of rotation red and green are not the same, the black arm must increase its length when it turns, I drawn a cylinder for that. 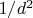The law of attraction of a spring is $1/d^2$ with d the distance from the red center. Note, the springs never lost any potential energy because the balls keep their position in the half disk.

The force F1 come from the pressure on the red wall.
The force F2 come from the pressure on the black center (the pressure to the half circle goes to the black center).
The force F3 come from the springs.
The torque T1 come from F1 around the red center.
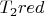
The torque T2 come from F2 around the black center. I calculated $T_2red$ too to verify the sum of torque around the red center is well at 0.

The device turns like that:



I calculated the forces and the torques around the red center, I'm not sure about F3x and F3y:

I don't take in account the sign of the value

$F_1 = \int_0^1 0.5 - \frac{1}{2-x} dx = 0.193147$

$F_2x = \int_{-\pi/2}^{\pi/2} 0.5-\frac{1}{\sqrt{(0.5\cos(x))^2+(1.5+1.5\sin(x))^2}}0.5\cos(x) dx = 1/6$

$F_3 = \int_{-\pi/2}^{\pi/2} 0.5-\frac{1}{\sqrt{(0.5\cos(x))^2+(1.5+1.5\sin(x))^2}}0.5\sin(x) dx = 0.182355$

$T_1 = \int_0^1 (0.5 - \frac{1}{2-x})(x-2) dx = 0.25$

$T_2red = 1/6 \cdot 1.5 = 0.25$

$F_3x = \int_{-\pi/2}^{\pi/2} \int_{0}^{0.5} \frac{\cos(\arctg(\frac{1.5+x\sin(y)}{x\cos(y)}))}{4(x\cos(y))^2 + (1.5+x\sin(y))^2} dxdy = 0.0264$

$F_3y = \int_{-\pi/2}^{\pi/2} \int_{0}^{0.5} \frac{\sin(\arctg(\frac{1.5+x\sin(y)}{x\cos(y)}))}{4(x\cos(y))^2 + (1.5+x\sin(y))^2} dxdy = 0.18221$




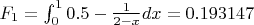
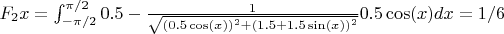
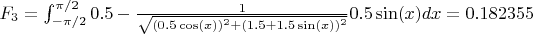
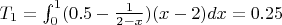
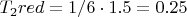
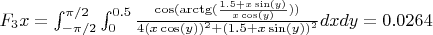
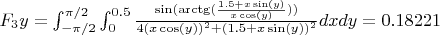
The sum of forces is well at 0. The sum of torques around the red center is well at 0.

The torque T2 around the black center and the energy:

I noted $A$ is the angle of rotation of the red arm (clockwise), in this case the black arm turns of $A/2$

$Fx=1/6

$Fy=\int_{-\pi/2}^{\pi/2} (0.5 - 1/\sqrt{ 0.5 \cos(x) + (1.5 + 0.5\sin(x) ) }) \sin(x) 0.5 dx = 0.182355$

$L=\sqrt{ (1.5 \cos(2 x))^2+(1.5+1.5 \sin(2x))^2}$
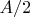
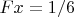
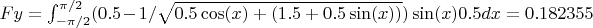
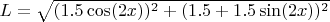
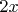
I need to have $2x$ inside trigo functions because when I integrate I do from 0 to $A/2$

$F=\sqrt{ Fx^2 + Fy^2 }$

$B=\arctg( Fx / Fy )$

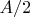
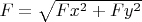
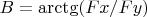
Torque $T_2$ around the black center:
$T_2= F L \sin( \pi/4 -x + B )$

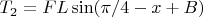
Energy recover from black arm:
$\int_0^{A/2} F L \sin( \pi/4 -x + B ) dx$

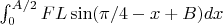
Energy needed to increase the length of the black arm:
$\int_0^{A/2} F L \cos( \pi/4 -x + B ) dx$

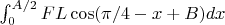
Energy needed from red arm:
$A\int_0^1 ( 0.5 - 1/(2-x) ) (x-2) dx$

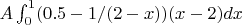
The sum of energy for an angle A:
$\int_0^{A/2} F L ( \sin( \pi/4 - x + B ) - \cos( \pi/4 - x + B ) ) dx - A\int_0^1 ( 0.5 - 1/(2-x) ) (x-2) dx$

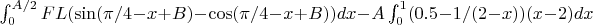
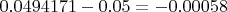
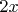
But the result is not 0, it is $0.0494171 - 0.05 = -0.00058$. The difference is small but it is not 0 because the integration take the force with a factor $\sin(x)-\cos(x)$ but the length change with square root of $2x$ . So, I think I made a mistake about an angle. If you can help me please ?


++

[Pphantom]

Тема перемещена из форума «Физика» в форум «Карантин»
по следующим причинам:

- неправильно набраны формулы (краткие инструкции: «Краткий FAQ по тегу [math]» и видеоролик Как записывать формулы);

Исправьте все Ваши ошибки и сообщите об этом в теме Сообщение в карантине исправлено.
Настоятельно рекомендуется ознакомиться с темами Что такое карантин и что нужно делать, чтобы там оказаться и Правила научного форума.

[Pphantom]

Тема перемещена из форума «Карантин» в форум «Помогите решить / разобраться (Ф)»

[Ludovic]

I don't wrote but there are 2 bodies:

1/ The red wall and its red arm
2/ The half disk and the black arm

If I let the device like that, the red wall rotates counterclockwise and the half disk rotates clockwise, the balls move closer to the red center and the springs lost their potential energy. But the springs keep their potential energy, so the balls don't move inside the half disk and sure I need to force the red wall to rotate clockwise. I calculated the energy I need to force the red wall and I calculated the energy I recover from the black arm less the energy I need to give for increase the length of the black arm.

Maybe it's easier to understand. It's a theoretical problem, imagine balls like molecules of water to have the pressure.

[Ludovic]

Sorry, I wrote a mistake in my Latex formulas, not in the drawing but only in the Latex:

$F_2x = \int_{-\pi/2}^{\pi/2} 0.5-\frac{1}{\sqrt{(0.5\cos(x))^2+(1.5+0.5\sin(x))^2}}0.5\cos(x) dx = 1/6$

$F_2y = \int_{-\pi/2}^{\pi/2} 0.5-\frac{1}{\sqrt{(0.5\cos(x))^2+(1.5+0.5\sin(x))^2}}0.5\sin(x) dx = 0.182355$

This don't change the difference of the result.

And maybe I made a mistake about $F_2y$, with a program I found $0.14709$. So, event it is that, the sum of energy is no 0. I found:

$F_2y=0.1409$

$B=\arctg( 1/6 / 0.14709 )$

The sum of energy for an angle A:
$\int_0^{A/2} F L ( \sin( \pi/4 - x + B ) - \cos( \pi/4 - x + B ) ) dx - A\int_0^1 ( 0.5 - 1/(2-x) ) (x-2) dx$



Like I computed this force I can't give the Latex equation but may it is only a problem of division about 2 and $\pi$. I will try to find.

[Ludovic]

Hi,

I think my integrals are good, I had an error in my program. So maybe someone knows where is my error ? Or at least tell me if the force F2y is correct or not.

Have a good day

[Ludovic]

I found now the same results with my programs and the integrals. I found the same results with the integrals and for an extremely low value (formula). So, the results in my first message are correct (image)

I drawn the details of the black axis, to show the semicircle can only turn around itself. I drawn 2 spokes but there are not in the same plan like that balls don't interact with the spokes. The semicircle can't have a torque around the black axis because it is a part of a circle and the forces of pressure are perpendicular to the surface: in theory I imagine the balls like molecules of water to use the same laws than fluids.



Integrals:

$F_1 = \int_0^1 0.5 - \frac{1}{2-x} dx = 0.193147$

$F_2x = \int_{-\pi/2}^{\pi/2} (0.5-\frac{1}{\sqrt{(0.5\cos(x))^2+(1.5+1.5\sin(x))^2}})0.5\cos(x) dx = 1/6$

$F_3 = \int_{-\pi/2}^{\pi/2} (0.5-\frac{1}{\sqrt{(0.5\cos(x))^2+(1.5+1.5\sin(x))^2}})0.5\sin(x) dx = 0.182355$

$T_1 = \int_0^1 (0.5 - \frac{1}{2-x})(x-2) dx = 0.25$

$T_2red = 1/6 \cdot 1.5 = 0.25$

$F_3x = \int_{-\pi/2}^{\pi/2} \int_{0}^{0.5} \frac{\cos(\arctg(\frac{1.5+x\sin(y)}{x\cos(y)}))}{4(x\cos(y))^2 + (1.5+x\sin(y))^2} dxdy = 0.0264$

$F_3y = \int_{-\pi/2}^{\pi/2} \int_{0}^{0.5} \frac{\sin(\arctg(\frac{1.5+x\sin(y)}{x\cos(y)}))}{4(x\cos(y))^2 + (1.5+x\sin(y))^2} dxdy = 0.18221$

I noted $A$ is the angle of rotation of the red arm (clockwise), in this case the black arm turns of $A/2$ The angle start at the vertical (0°).

$Fx=1/6

$Fy=\int_{-\pi/2}^{\pi/2} (0.5 - 1/\sqrt{ 0.5 \cos(x) + (1.5 + 0.5\sin(x) ) }) \sin(x) 0.5 dx = 0.182355$

$L=\sqrt{ (1.5 \cos(2 x))^2+(1.5+1.5 \sin(2x))^2}$
I need to have $2x$ inside trigo functions because when I integrate I do from 0 to $A/2$

$F=\sqrt{ Fx^2 + Fy^2 }$

$B=\arctg( Fx / Fy )$

Torque $T_2$ around the black center:
$T_2= F L \sin( \pi/4 -x + B )$

Energy recover from black arm:
$\int_0^{A/2} F L \sin( \pi/4 -x + B ) dx$

Energy needed to increase the length of the black arm:
$\int_0^{A/2} F L \cos( \pi/4 -x + B ) dx$

Energy needed from red arm:
$A\int_0^1 ( 0.5 - 1/(2-x) ) (x-2) dx$

The sum of energy for an angle A:
$\int_0^{A/2} F L ( \sin( \pi/4 - x + B ) - \cos( \pi/4 - x + B ) ) dx - A\int_0^1 ( 0.5 - 1/(2-x) ) (x-2) dx$

But the result is not 0, from 0 to 0.1 rd it is $0.0494171 - 0.05 = -0.00058$. The difference from 0 to 0.5 rd is $0.25-0.219 = 0.031$ it is 12.5% of the greater value.

I have the definite integrals for all integrals so it's not possible to a problem of accuracy. So maybe a problem with an angle ?

Maybe the image with a small rotation don't explain how axis works:

The red wall is free to rotate around the fixed red axis (red axis is fixed to the ground). A motor force the red wall to rotate clockwise and follow the half disk. The balls never lost their position in the half disk.
The black arm (telescopic) is free to rotate around the fixed green axis (green axis is fixed to the ground), it follows the half disk because it has the force F2 on the black axis
The semicircle is free to rotate around the black dot but it don't rotate around it because there is no torque (in theory)

[Ludovic]

Nobody ? It's difficult to understand the device ? I can explain or draw another views, tell me.

[arbuz]

Ludovic писал(а):

It's difficult to understand the device ?

Чиво-чиво? Если вы поставили знак вопроса, то надо переставить местами It и is... Получится "Is it difficult ... ?"
После исрпавления ряда явных ошибок ваш текст может получить статус "Russian English" по терминологии редакторов англо-американских журналов.

[Ludovic]

yes, is it difficult ?
"Russian English" I take this like комплимент

[arbuz]

Это ваше право принимать мои слова (Russian English) за комплимент, но для редакторов это обычно является причиной отфутболивания статьи.

[Pphantom]

arbuz, полезно учитывать, что если кто-то не владеет русским языком, то отсюда не следует, что английским тот же кто-то владеет как родным. Противоположное обратному утверждение, соответственно, также неверно.

[Ludovic]

I'm not english. I do what I can :/

[Ludovic]

Tell me what you don't understand, please.

[Ludovic]

I change the integrals of $F_3$ because I divided by 4 to have the good value but the true integrals are:

$F_3x = \int_{-\pi/2}^{\pi/2} \int_{0}^{0.5} x\frac{\cos(\arctg(\frac{1.5+x\sin(y)}{x\cos(y)}))}{(x\cos(y))^2 + (1.5+x\sin(y))^2} dxdy = 0.0264$

$F_3y = \int_{-\pi/2}^{\pi/2} \int_{0}^{0.5} x\frac{\sin(\arctg(\frac{1.5+x\sin(y)}{x\cos(y)}))}{(x\cos(y))^2 + (1.5+x\sin(y))^2} dxdy = 0.182$

The results don't change (In the contrary the sum of forces is not 0). Note $dx$ is the radius and $dy$ the angle with polar coordinates.