сходимость средних статистических оценок

[ecartman]

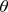
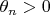
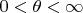
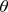
Пусть $\theta_n$ есть некая статистическая оценка параметра $\theta$. Известно, что $\theta_n>0$ п.н. и что $0<\theta<\infty$. Известно также что $\theta_n$ сходится по вероятности к $\theta$ с ростом $n$, и что $E[\theta_n]\to\theta$ при $n\to\infty$.

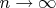
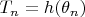
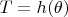
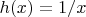
Пусть теперь $T_n=h(\theta_n)$ и $T=h(\theta)$, где $h(x)=1/x$. Требуется доказать что $EV[T_n/T]\to1$ при $n\to\infty$.
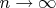
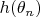
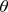
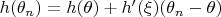
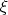
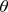
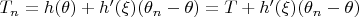
Цепь рассуждений следующая: разложим $h(\theta_n)$ в ряд Тейлора в окрестности точки $\theta$, т.е. $h(\theta_n)=h(\theta)+h'(\xi)(\theta_n-\theta)$, где $\xi$ лежит между $\theta_n$ и $\theta$. Тогда имеем: $T_n=h(\theta)+h'(\xi)(\theta_n-\theta)=T+h'(\xi)(\theta_n-\theta)$ и

$$ \frac{T_n}{T}=1+\frac{h'(\xi)(\theta_n-\theta)}{T}. $$

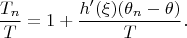
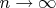
Осталось показать, что $E[h'(\xi)(\theta_n-\theta)]\to0$ при $n\to\infty$. Пытаюсь разбить это мат ожидание на две части: $E[h'(\xi)(\theta_n-\theta)]=E[h'(\xi)(\theta_n-\theta);\theta_n>\theta]+E[h'(\xi)(\theta_n-\theta);\theta_n<\theta]$. Корректно ли сказать, что $E[h'(\xi)(\theta_n-\theta);\theta_n>\theta]\le0$ и соответственно $E[h'(\xi)(\theta_n-\theta)]\le E[h'(\xi)(\theta_n-\theta);\theta_n<\theta]$ и далее, что т.к. 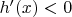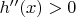$h'(x)<0$ и $h''(x)>0$, то $E[h'(\xi)(\theta_n-\theta);\theta_n<\theta]\le h'(\theta)E[\theta_n-\theta;\theta_n<\theta]$?

И можно ли утверждать, что $E[\theta_n-\theta;\theta_n<\theta]\to0$ при $n\to\infty$?

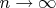
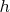
Меня на самом деле интересует более сложная функция $h$, но ее поведение суть мало чем отличается от $1/x$.
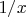
Спасибо за помощь.

[--mS--]

Пусть, например, $X_i$ из нормального распределения со сдвигом $\theta$ и единичной дисперсией, $\theta_n=\overline X$, $\mathsf E\theta_n=\theta$. Тем не менее $\mathsf E\dfrac{1}{\theta_n}$ не существует.

[ecartman]

--mS-- в сообщении #923033 писал(а):

Пусть, например, $X_i$ из нормального распределения со сдвигом $\theta$ и единичной дисперсией, $\theta_n=\overline X$, $\mathsf E\theta_n=\theta$. Тем не менее $\mathsf E\dfrac{1}{\theta_n}$ не существует.

Но в этом случае не будет иметь место $\bar{X}_n>0$ п.н.

А если в моем вопросе наложить условие, что $E[|\theta_n|]<\infty$, можно ли тогда утверждать что $E[T_n/T]\to0$ при $n\to\infty$?

[--mS--]

Ну, подумаешь, возьмите модули. Возьмите неотрицательные величины - например, равномерные на $[0, 2\theta]$ вместо нормальных. В любом случае математическое ожидание $1/\theta_n$ не существует.

(Оффтоп)

Сходимость последовательности математических ожиданий сходящейся (слабо или по вероятности, не суть) последовательности случайных величин равносильна равномерной интегрируемости этой последовательности. Что бы и как бы Вы ни доказывали в общих условиях, в любом случае всё сведётся к проверке равномерной интегрируемости.

-- Вс окт 26, 2014 21:17:44 --

ecartman в сообщении #923131 писал(а):

А если в моем вопросе наложить условие, что $E[|\theta_n|]<\infty$, можно ли тогда утверждать что $E[T_n/T]\to0$ при $n\to\infty$?

Вы и так предположили, что матожидания существуют, это никак не спасает от отсутствия матожидания у обратных величин.

[ecartman]

--mS-- в сообщении #923177 писал(а):

Вы и так предположили, что матожидания существуют, это никак не спасает от отсутствия матожидания у обратных величин.

Да, конечно. А если предположить, что оценка такая, что мат ожидание от обратной величины существует?

[--mS--]

Тогда уж лучше сразу предположить, что $\mathsf E\dfrac{1}{\theta_n}\to\dfrac{1}{\theta}$, и вся недолга. Предположения обычно накладывают на исходные объекты.

Впрочем, какая разница? $\mathsf P(\xi_n=1)=1-\dfrac1n=1-\mathsf P\left(\xi_n=\dfrac1n\right)$. Проверьте, что $\xi_n\stackrel{p}{\to} 1$, $\mathsf E\xi_n \to 1$, но $\mathsf E\dfrac{1}{\xi_n}\to 2$. Несмотря на то, что $\dfrac{1}{\xi_n}\stackrel{p}{\to} 1$ и их матожидания вполне себе существуют.

Вам бы почитать в учебниках о сходимостях случайных величин и их матожиданий.