Какая-то тревожная последовательность вопросов. Например, пришёл человек, спрашивает про разведение тигров. Деловито записал все подробности - про сырое мясо и т.д. Под конец случайно выясняется, что он считает тигров разновидностью бабочек.
Также у меня рождаются сомнения, правильно ли я понял, что такое
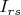
. А что это такое, really? Написано красиво, спору нет, но... не лучше ли сказать
словами?
Слова автора учебника(мне с этого трудно сделать какие либо выводы):
Let
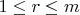
and
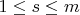
. Let
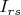
be the square
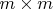
matrix which has component
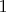
in the
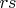
place, and

elsewhere.
Let A =

be any
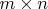
matrix. What is the effect of multiplying
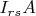
?
The definition of multiplication of matrices shows that
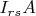
is the matrix
obtained by putting the

-th row of

in the

-th row, and zeros elsewhere.
If

then
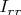
has a component
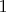
on the diagonal place, and

elsewhere. Multiplication by
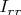
then leaves the

-th row fixed, and replaces
all the other rows by zeros.
If
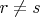
let
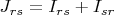
Then
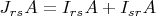
Then
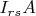
puts the

-th row of

in the

-th place, and
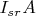
puts the

-th row of

in the

-th place. All other rows are replaced by zero.
Thus
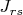
interchanges the

-th row and the

-th row, and replaces all
other rows by zero.
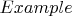
. Let

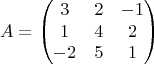
If you perform the matrix multiplication, you will see directly that
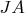
interchanges the first and second row of

, and replaces the third row by zero.
.