Let
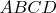
is a convex quadrilateral.
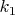
and
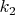
are the circumcircles of the triangles

and
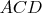
.
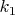
intersects segments
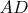
and
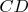
at the points
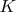
and
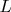
.
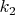
intersects segments

and

at the points

and
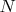
.

is the intersection point of

and

.
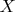
is the intersection point of

and
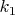
.
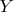
is the intersection point of

and
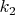
.
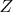
and
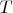
are the intersection points of

and

with
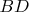
, respectively. Prove that
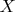
,
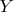
,
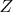
,
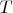
are concyclic.