Сигмоидные функции

[zodiac]

Даны две функции:
$ \phi(v) = \frac{1}{\sqrt{2\pi}}\int_{-\infty}^{v}exp(-\frac{x^2}{2})dx $
$ \phi(v) = \frac{2}{\pi}arctg(v) $
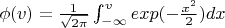
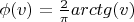
Необходимо обосновать, что они обе подходят под определение сигмоидной функции.
Более-менее определение нашел в википедии:

Цитата:

For lack of complex descriptions a sigmoid function is often used. A sigmoid curve is produced by a mathematical function having an "S" shape.
...
In general, a sigmoid function is real-valued and differentiable, having either a non-negative or non-positive first derivative and exactly one inflection point. There are also a pair of horizontal asymptotes as $ t \to \pm \infty $

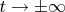
Как я понял, необходимо доказать, что данные функции дифференцируемы (найти предел?), первую производную и точку перегиба. Построить график для того, чтобы убедиться, что он S-образный, а так же найти горизонтальные асимптоты.
Правильно?

[Хорхе]

Надо доказать, что производные обеих функций сохраняют знак, что у каждой единственная точка перегиба и две горизонтальные (именно так переводится слово "horizontal" на русский) асимптоты.

[ewert]

кто бы ещё объяснил, кому и зачем эти "сигмоиды" могут быть любопытны?... Ну мало ли какое возможно сочетание монотонностей и выпуклостей...

[zodiac]

Хорхе в сообщении #285266 писал(а):

горизонтальные

Ну конечно же горизонтальные, небольшая опечатка вышла:)

ewert в сообщении #285271 писал(а):

кто бы ещё объяснил, кому и зачем эти "сигмоиды" могут быть любопытны?... Ну мало ли какое возможно сочетание монотонностей и выпуклостей...

В нейронных сетях как функция активации.