Bridgeport писал(а):
I found trivial answer to the first question:
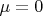
. The second question I think now is easy
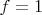
.
Any non-trivial solutions?.....
P.S I just edited original message. I added in the first question: for all
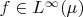
?
Now I see that suggestion to try

is similar to trivial solution
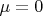
( after fixing initial typos.)
Sorry for so much confusion!
Well, the problem actually is not that easy.
I was
not suggesting that you take

on whole domain, say

. Only on some subset
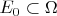
with
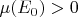
. Then all functions that have
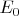
as support will be mapped into zero. Which means they are not in the range of
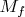
and therefore,
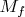
is not ONTO. So throw such an

from
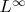
because it does not satisfy the requirement.
You were right about
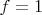
but it's just a particular case.
My main idea was: by definition the map
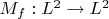
is onto, if for any
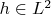
there is a preimage
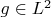
such that
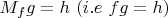
. But as long as

stays away from zero on it's domain, you can always solve that equation for
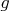
just by dividing both sides by

i.e.
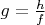
. However this is sufficient condition. May be not necessary. Try to play with the rest.
Bridgeport писал(а):
Question: for which measure
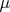
is it true that
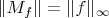
for all
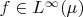
?
Not sure what is being asked here. I couldn't find this problem in my Rudin's book. Perhaps different edition, I guess.
Anyway, the solution
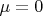
seems wrong. We need to figure for which set function
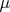
the following is true:
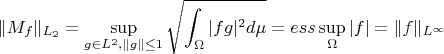
for all

It is hard to answer without knowing the context of the problem.