Люди, чё думаете, связаны эти классы универсальности с классами вычислительной сложности?
arxiv.org/abs/cs/0608053
Renormalization group approach to the P versus NP question
S.N. Coppersmith
This paper argues that the ideas underlying the renormalization group technique used to characterize phase transitions in condensed matter systems could be useful for distinguishing computational complexity classes. The paper presents a renormalization group transformation that maps an arbitrary Boolean function of
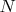
Boolean variables to one of
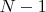
variables. When this transformation is applied repeatedly, the behavior of the resulting sequence of functions is different for a generic Boolean function than for Boolean functions that can be written as a polynomial of degree
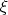
with
as well as for functions that depend on composite variables such as the arithmetic sum of the inputs. Being able to demonstrate that functions are non-generic is of interest because it suggests an avenue for constructing an algorithm capable of demonstrating that a given Boolean function cannot be computed using resources that are bounded by a polynomial of
.
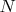
