Problem: Consider 3 mass distributions in the plane that moreover, assign measure 0 to each circle. Prove that they can be simultaneously halved (bisected) by a circle or by a straight line. (This is a special case of rezults of Stone and Tukey; see G. Bredon.
Topology and Geometry. Graduate Texts in Mathematics 139. p. 243)
I have a
hint: Map the sets into
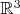
by the mapping
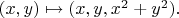
How is halving of the sets by circle
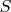
in the plane related to dissection of their images in
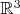
by planes?
Of course, a
Ham sandwich theorem for measures should be used (something like this): when in
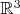
by a plane we do bisection of images of those 3 mass distributions (sets), than intersection of that plane and paraboloid

is ellipse. How to find correspondence of that ellipse and circle which bisects those 3 mass distributions in plane (
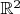
)?
Thanks in advance.