System of equations from an old magazine

[ins-]

Solve the system:
$1 + (y-z)^2 = 2 x$
$16 + (z-x)^2 = 8 y$
$81 + (x-y)^2 = 18 z$

[nnosipov]

Maple says that there are infinitely many solutions (for instance, over $\mathbb{R}$). I propose solve it over $\mathbb{F}_p$ with a prime $p>3$.

Upd. For the number of solutions over $\mathbb{F}_p$, we have a very simple answer. So, this problem can be proposed for student's exam tests. In the form when the coefficient $16$ was replaced by $17$ (due to a teacher's errata, for example) the problem seems to be more surprising but still reasonable.

[Rak so dna]

ins- в сообщении #1474253 писал(а):

Solve the system:
$1 + (y-z)^2 = 2 x$
$16 + (z-x)^2 = 8 y$
$81 + (x-y)^2 = 18 z$

$\begin{cases} -3 + (y-z)^2 - \frac{(z-x)^2}{4} = 2(x-y),&\\ -5 + \frac{(z-x)^2}{4} - \frac{(x-y)^2}{9} = 2(y-z) \end{cases}$
складывая это, получим:

$9(y-z-1)^2=(x-y+9)^2$
отсюда:

$x=13, y=10, z=5$ или
$x=\frac{p^2+1}{2}, y=\frac{p^2+6p+13}{2}, z=\frac{p^2+4p+13}{2}$.

[ИСН]

Curiouser and curiouser! A whole parabola of solutions in $\mathbb Z$ alone (that is, as $p$ runs over all odd integers from $-\infty$ to $\infty$).

[ins-]

It should not be a hard problem, but it is possible to make a mistake while solving it. That is the reason I think it is suitable for lower rounds of a math olympiad. I found the problem in an old magazine from Sweden (1942) as a statement (there was no solution). I made some attempts to solve it.

From the first equation we have $\frac{1+(y-z)^2}{2}=x$ *)
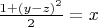
From the second equation we have $\frac{16+(z-x)^2}{8}=y$ **)
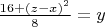
Then from *) - **) we have:
$\frac{1+(y-z)^2}{2}-\frac{16+(z-x)^2}{8}=x-y$
$\frac{1+(y-z)^2}{2}-\frac{16+(z-x)^2}{8}=(x-z)+(z-y)$
$\frac{1+(y-z)^2}{2}-\frac{16+(z-x)^2}{8}=-(z-x)-(y-z)$
$\frac{1+(y-z)^2}{2}+(y-z)=\frac{16+(z-x)^2}{8}-(z-x)$
$\frac{(1+(y-z))^2}{2}=\frac{(4-(z-x))^2}{8}$ ***)

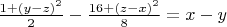
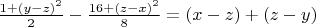
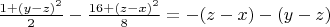
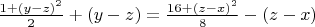
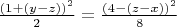
Or we have two cases:
$I. $ $2(1+y-z)=4-z+x$
$II. $ $2(1+y-z)=-4+z-x$

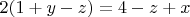
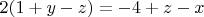
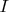
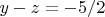
In case $I$ there are two options: $y-z = -5/2$ or $y-z=2$.
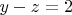
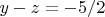
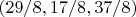
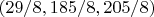
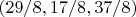
When $y-z=-5/2$ I found the following possible solutions $(29/8, 17/8, 37/8)$ and $(29/8, 185/8, 205/8)$ but when tried to put these values in the system's equations it turned out that the second system equation is not an identity, so only $(29/8, 17/8, 37/8)$ is a solution, when $y-z=-5/2$.
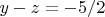
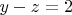
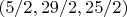
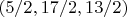
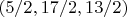
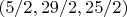
When $y-z=2$ I found the following possible solutions $(5/2, 29/2, 25/2)$ and $(5/2, 17/2, 13/2)$, but again the second equation is not an identity for $(5/2, 17/2, 13/2)$, so only $(5/2, 29/2, 25/2)$ is a solution when $y-z=2$.

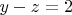
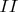
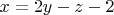
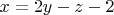
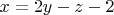
In case $II$ I found $x=2y-z-2$. When I tried to plug x in the system's equations I had an identity. It made me to think that the system have in this case an infinite number of solutions given by the condition: $x=2y-z-2$. To verify it is correct I tried to put some solutions of $x=2y-z-2$ in the system's equations, but it they weren't an identities.

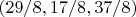
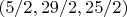
So I concluded - only the triples $(29/8, 17/8, 37/8)$ and $(5/2, 29/2, 25/2)$ are the solutions of the system.

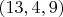
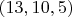
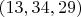
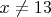
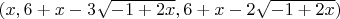
But ... I put the system in Wolfram Alpha and it gave a very different result: $(13, 4, 9)$, $(13, 10, 5)$, $(13, 34, 29)$ and when $x \ne 13$ there are infinitely many solutions given by: $(x, 6 + x - 3 \sqrt{-1 + 2 x}, 6 + x - 2 \sqrt{-1 + 2 x})$ and $(x, 6 + x + 3 \sqrt{-1 + 2 x}, 6 + x + 2 \sqrt{-1 + 2 x})$.

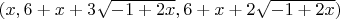
Probably I made a mistake or it needs more efforts in solving. Still working on it.

[kotenok gav]

ins- в сообщении #1474328 писал(а):

In case $I$ there are two options: $y-z = -5/2$ or $y-z=2$.

Why?

[ins-]

kotenok gav в сообщении #1474329 писал(а):

ins- в сообщении #1474328 писал(а):

In case $I$ there are two options: $y-z = -5/2$ or $y-z=2$.

Why?

Because of the following:

I put $y-z=t$ after some transformations using the second and third system's equations there was the following:

$\frac{16+(2t+2)^2}{8}-\frac{81+(t-2)^2}{18}=t$. I solved it and it has two roots $-\frac{5}{2}$ and $2$.

In case it is not clear I can provide more details.

[Rak so dna]

ins- в сообщении #1474328 писал(а):

In case $II$ I found $x=2y-z-2$. When I tried to plug x in the system's equations I had an identity. It made me to think that the system have in this case an infinite number of solutions given by the condition: $x=2y-z-2$. To verify it is correct I tried to put some solutions of $x=2y-z-2$ in the system's equations, but it they weren't an identities.

A typical situation is when "necessity" and "sufficiency" are confused.

ins- в сообщении #1474328 писал(а):

But ... I put the system in Wolfram Alpha and it gave a very different result: $(13, 4, 9)$, $(13, 10, 5)$, $(13, 34, 29)$ and when $x \ne 13$ there are infinitely many solutions given by: $(x, 6 + x - 3 \sqrt{-1 + 2 x}, 6 + x - 2 \sqrt{-1 + 2 x})$ and $(x, 6 + x + 3 \sqrt{-1 + 2 x}, 6 + x + 2 \sqrt{-1 + 2 x})$.

in particular when $x=\frac{p^2+1}{2}$ that's exactly

Rak so dna в сообщении #1474317 писал(а):

$x=13, y=10, z=5$ или
$x=\frac{p^2+1}{2}, y=\frac{p^2+6p+13}{2}, z=\frac{p^2+4p+13}{2}$.

Answers $(13, 4, 9)$, $(13, 34, 29)$ are achieved at $p=\mp5$
Sorry for my English

[ins-]

Thank you for these insights!

Rak so dna в сообщении #1474334 писал(а):

Sorry for my English

Your English is far better than my Russian. It is a sad story I haven't used it for many years and write here in English.

The problem allows more approaches to be solved. For example $2a=x+y-z$, $2b=x-y+z$, $2c=-x+y-z$, but I not sure which one is the best.

[nnosipov]

ins-
How about my idea for solving your system over a finite field (see above)?

[ins-]

It is very interesting and in this way can be created many problems. I think many of the good problems are created in the way "what will happens if we change this to that". Somewhere I found a material where was mentioned the famous Russian mathematician Sharygin created some of his problems using exactly that approach.

[nnosipov]

At least, I constructed (with your help, of course) these two problems for my students which will get it in the nearest exam session

[ins-]

I hope they read this forum.

[nnosipov]

They read, I know.

[ins-]

You are lucky having such ambitious students.