Второй дифференциал всё-таки инвариантный?

[StaticZero]

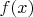
Существо проблемы вот в чём. Пусть есть функция $f(x)$. Возьмём от неё полное приращение:

$$ \mathrm df = f'_x \ \mathrm dx. \eqno (1) $$

Теперь возьмём полное приращение второго порядка:

$$ \mathrm d^2 f = f''_{xx} \ \mathrm dx^2. \eqno(2) $$

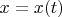
Теперь изменим ситуацию и параметризуем аргумент: $x = x(t)$. Как известно, в таком случае

$$ \mathrm df = f'_x (x(t)) \dot x \ \mathrm dt. \eqno(3) $$

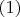
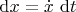
Это равенство эквивалентно $(1)$, если в него подставить $\mathrm dx = \dot x \ \mathrm dt$. Теперь попробуем написать второе равенство:

$$ \mathrm d^2 f = \mathrm d \left( \dfrac{\mathrm df(x(t))}{\mathrm dx} \cdot \dfrac{\mathrm dx}{\mathrm dt}\right) \ \mathrm dt = \mathrm d\left(\dfrac{\mathrm df(x(t))}{\mathrm dx}\right) \dot x \ \mathrm dt + \mathrm d\left(\dfrac{\mathrm dx}{\mathrm dt}\right) \cdot f'_x(x(t)) \ \mathrm dt = \ldots \eqno\left(4 \frac{1}{2}\right) $$

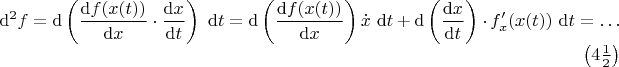
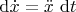
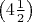
Здесь очевидно, что $\mathrm d \dot x = \ddot x \ \mathrm dt$. Для того, чтобы найти $\mathrm d(f'_x[x(t)])$, используем правило о сложной функции: $\mathrm d(f'_x[x(t)]) = f''_{xx} \dot x \ \mathrm dt$. Постановка этих двух равенств в $\left(4 \frac{1}{2}\right)$ даёт окончательно равенство $(4)$:

$$ \ldots = \mathrm d^2 f = f''_{xx} \dot x^2 \ \mathrm dt^2 + f'_x \ddot x \ \mathrm dt^2 = \Big(f''_{xx} \dot x^2 + f'_x \ddot x \big) \ \mathrm dt^2. \eqno(4) $$

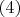
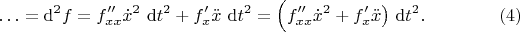
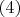
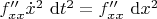
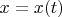
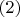
Легко видеть, что в среднюю часть $(4)$ входит слагаемое вида $f''_{xx} \dot x^2 \ \mathrm dt^2 = f''_{xx} \ \mathrm dx^2$. Это то слагаемое, которое получается при подстановке $x = x(t)$ в $(2)$. Утверждение о "неинвариантности второго дифференциала" говорит о том, что так делать нельзя.

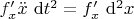
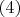
Однако, второе слагаемое имеет вид $f'_x \ddot x \ \mathrm dt^2 = f'_x \ \mathrm d^2 x$. Всю вместе формулу $(4)$ можно формально переписать в виде

$$ \mathrm d^2 f = f''_{xx} \ \mathrm dx^2 + f'_x \ \mathrm d^2 x, \eqno(5) $$

который получается и из других соображений:

$$ \mathrm d^2 f = \mathrm d(\mathrm df) = \mathrm d(f'_x \ \mathrm dx) = \mathrm df'_x \ \mathrm dx^2 + f'_x \ \mathrm d(\mathrm dx) = f''_{xx} \ \mathrm dx^2 + f'_x \ \mathrm d^2 x, \eqno(6) $$

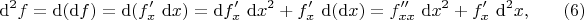
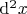
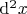
если положить, что в случае, когда $x$ свободная переменная, то $\mathrm d^2 x$ равен нулю, а если $x$ сам является функцией, то $\mathrm d^2 x$ нулю уже не равен.

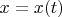
Так в чём состоит польза от утверждения, что второй дифференциал "не инвариантный"? Ведь если подставлять туда $x = x(t)$ в его правильную форму

$$ \mathrm d^2 f = f''_{xx} \ \mathrm dx^2 + f'_x \ \mathrm d^2 x, $$

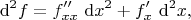
то всё становится на свои места.

[Someone]

Дык, неинвариантность и означает, что вместо формулы (2) получается (5). А польза этого предупреждения состоит в том, чтобы помнить его и понимать, когда можно пользоваться формулой (2), а когда нужно использовать более громоздкую формулу (5).

[StaticZero]

Someone в сообщении #1225816 писал(а):

Дык, неинвариантность и означает, что вместо формулы (2) получается (5). А польза этого предупреждения состоит в том, чтобы помнить его и понимать, когда можно пользоваться формулой (2), а когда нужно использовать более громоздкую формулу (5).

Угу. такой ответ меня полностью бы даже удовлетворил, за исключением одной детали, которая, скорее, относится к методу рассказывания этого всего хозяйства. Логичным кажется сначала получить (5), а (2) указывать в виде частного случая. Или к значку дифференциала дописывать переменную, по которой дифференциал берётся, чтобы аналогия с правилом Лейбница стала ещё более тесной.

[Someone]

StaticZero в сообщении #1225819 писал(а):

Логичным кажется сначала получить (5), а (2) указывать в виде частного случая.

Нет, не логичнее. Поверьте преподавателю с более чем сорокалетним стажем. Как раз очень естественно определить производные и дифференциалы сначала по независимой переменной, а потом уже разбираться с композициями функций и их производными и дифференциалами. При этом никаких новых определений уже не требуется, и формула (5) вытекает из формулы (2) и свойств этих самых композиций.

[StaticZero]

Someone в сообщении #1225821 писал(а):

Нет, не логичнее. Поверьте преподавателю с более чем сорокалетним стажем

Спорить не буду, вам, очевидно, виднее, да и в общем, согласен. Самому бы с этого начали объяснять — нифига бы не понял.