Выглядит достаточно прозрачно, но
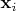
должны обязательно быть строками. Если это столбцы, их надо транспонировать, а если это «просто» векторы, то лучше бы получение компонент в каком-то базисе записать явно, иначе запись, строго говоря, некорректна. Впрочем, если
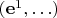
— базис, сопряжённый базису
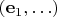
в
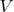
,
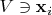
, компоненты в котором и даны:
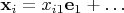
, то ваша матрица будет иметь и совершенно* корректную запись
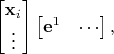
причём если
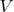
у нас со скалярным произведением, то мы, конечно, можем вместо
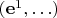
взять
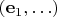
и умножать векторы скалярным произведением.
* К этой тоже можно придраться, но это труднее.