Not a complete solution at all, but might be of some interest: taking
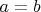
one gets

,
where

is a

degree polynomial with all positive coefficients; it can be found w/o computer, as it is just

divided by its easily seen divisor

. Now the hard part is to show that
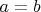
is the only extremum, not sure is there any natural way to complete this...