Помогите понять доказательство

[Neytrall]

Вот утверждение и его доказательство:

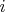
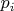
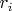
Let us suppose that probability of success for the treated individual $i$ is $p_i$, and $r_i$ for the untreated. Now, assume that equality
$\frac{p_i}{1-p_i}=\theta\frac{r_i}{1-r_i}$
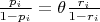
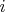
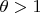
holds for all $i$ and for some $\theta>1$.Therefore:
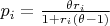
$p_i=\frac{\theta r_i}{1+r_i (\theta-1)}$ ,with $0<p_i<1$
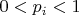
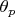
Now, let us define the pooled multiplier $\theta_p$ as
$\theta_p=\frac{p_a/(1-p_a)}{r_a/(1-r_a)}$
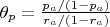
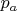
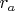
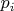
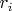
where $p_a$and $r_a$are average values of $p_i$ and $r_i$, respectively.

Proposition 1. Using the notation given above, the following inequality holds $\theta>\theta_p$
Proof. Suppose $\theta>1$.
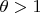
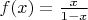
Let $f(x)=\frac{x}{1-x}$ for $x\in(0,1)$.
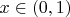
So $f$ is a strictly increasing function.
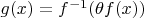
Let $g(x)=f^{-1} (\theta f(x))$, so $g(r_i )=f^{-1} (\theta f(r_i ))=f^{-1} (\theta \frac{r_i}{1-r_i})=f^{-1} (\farc{p_i}{1-p_i})=f^{-1} (f(p_i))=p_i$.
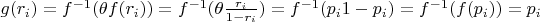
The inequality of Proposition 1, $\theta>\theta_p$ means that $f(p_a)<\theta f(r_a)$.
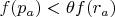
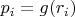
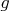
As it is shown above $p_i=g(r_i)$, so we can prove Proposition 1 by proving that function $g$ is strictly concave. But
$g(x)=\frac{\theta x}{1+(\theta-1)x}=\frac{\theta}{1-\theta}(1-\frac{1}{1+(\theta-1)x})$
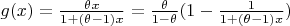
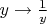
and $ y\to\frac{1}{y}$ is strictly convex for $y>0$.

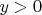
мне совершенно не понятна последняя часть:
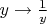
"and $ y\to\frac{1}{y}$ is strictly convex for $y>0$"
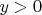
Можете мне её объяснить? Что это и почему это верно?

[Joker_vD]

"and $y\mapsto\frac1y$ is strictly convex for $y>0$", скорее всего. Ну пожалели люди букву: "and function $h(y)=\frac1y$ is strictly convex for $y>0$". Фраза "strictly convex for $y>0$" означает, что для любых различных $y_1,y_2>0$ выполнено неравенство

$$h(\mu y_1 + \nu y_2)<\mu h(y_1)+\nu h(y_2),\qquad 0\leqslant\mu,\nu\leqslant1,\;\mu+\nu=1.$$

[Neytrall]

Не уверен, это все таки научная статья.
К тому же, не понятно как это доказывает утверждение, ведь до этого было сказанно "we can prove Proposition 1 by proving that function $g$ is strictly concave", а здесь говорят об strictly convex.