Let
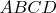
is a complete quadrilateral with intersection point of the diagonals -

.
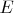
is the intersection point of

and
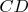
.
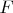
is the intersection point of
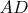
and
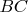
.
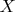
is a random point (outside triangle

).
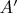
,

,
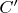
,
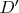
,

are the intersection points of

,

,

,

,

with
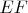
, respectively. Prove that:
a)

;
b)

.
(Оффтоп)
Greetings to Dimoniada :)