Let

and let

for all

.
Note that the intervals

are non-overlapping for all

. Also,

and

for all

For any

, we have:

For any n

, we have:

By the mean value theorem, there exists an

such that:

Clearly,

becomes arbitrarily large as
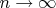
. Thus, selecting an
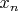
this way in every interval

yields a sequence of real numbers

such that

as desired