Жадность правит миром. Чего люди только не делают, чтобы получить миллион американских долларов. Даже доказывают, что P=NP.
Очередная попытка от Matt Groff называется: "Towards P = NP via k-SAT: A k-SAT Algorithm Using Linear Algebra on Finite Fields". Автор утверждает, что решит задачу выполнимости булевой функции за
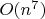
.
http://arxiv.org/abs/1106.0683Abstract:
The problem of P vs. NP is very serious, and solutions to the problem can help save lives. This article is an attempt at solving the problem using a computer algorithm. It is presented in a fashion that will hopefully allow for easy understanding for many people and scientists from many diverse fields.
In technical terms, a novel method for solving k-SAT is explained. This method is primarily based on linear algebra and finite fields. Evidence is given that this method may require only
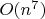
time and space for deterministic models. It’s concluded that significant evidence exists that P=NP.
There is a forum devoted to this paper at
http://482527.ForumRomanum.com. All are invited to correspond here and help with the analysis of the algorithm.
Самое любопытное, что после того как товарищ Vinay Deolalikar в прошлом году попал под раздачу, люди пытаются использовать его опыт и по собственной инициативе начинают публичное обсуждение своей работы. Автор создал отдельный форум, для обсуждения алгоритма! Вот он новый метод решения научных проблем в 21 веке.
