Points
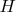
and
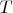
are marked respectively on the sides
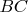
abd
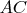
of triangle
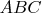
so that

is the altitude and

is the bisectrix
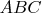
. It is known that the gravity center of
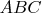
lies on the line

.
a) Find
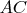
if
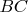
=a and

=c.
b) Determine all possible values of

for all triangles
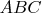
satisfying the given condition.